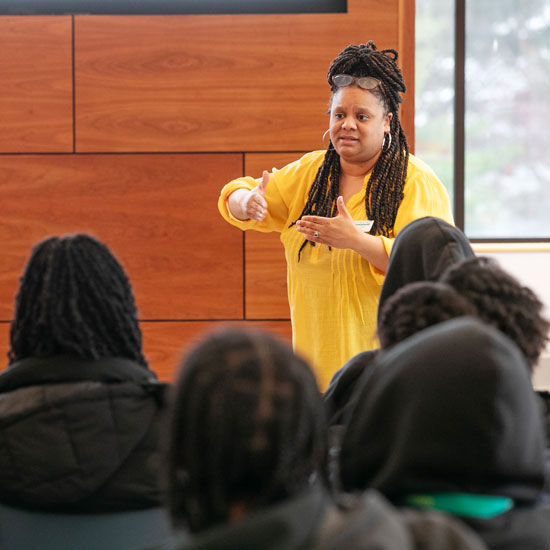Campus and Regional Events
Campus and Regional Events
- The Skidmore Opportunity Program’s director discusses how OP listens to students' needs and helps them grow and thrive.
- The College is joining 60 other college presidents of diverse institutions from across the country to advance higher education’s pivotal role in preparing students to be engaged citizens and to uphold free expression on campus.
- An abundance of lectures, performances, and athletic events has campus buzzing about a spring semester that is truly difficult to eclipse.
Thought
Matters
We believe that every life is made more profound with creativity at its core.
Read MoreIt's something we hear often: Students come to Skidmore to "do both." They want to explore multiple passions – science and music, performance and politics. They seek the freedom, encouragement, and support to explore, mix, match, add, and create. We believe we offer all of this and more ... but we'll let them show and tell you themselves.